PLASMA (Greek πλάσμα - sculpted, shaped), ionized gas consisting of electrons and ions, the movement of which is determined primarily. the collective nature of the interaction due to long-range electromagnetic forces, in contrast to ordinary gas, in which short-range pair interactions (collisions) dominate. P.'s high electrical conductivity makes it sensitive to the effects of electromagnetic fields. The specificity of P.'s response to such an influence allows us to consider P. a special (fourth) state of aggregation of matter, along with solids, liquids, and gases.
Basic parameters and properties of plasma
Quantitatively, P. is characterized by the concentrations of electrons $n_e$ and ions $n_i$, their cf. temperatures (energies) $T_e$ and $T_i$, degree of ionization (fraction of ionized atoms) $α=n_i/(n_i+n_0)$, where $n_0$ is the concentration of neutral atoms, cf. charge of the ion $Z_{eff}$. The high mobility of plasma particles (especially electrons) ensures screening of the charge introduced into the plasma at distances on the order of the Debye screening radius $r_D$ for times on the order of the inverse plasma electron (Langmuir) frequency, $ω_{re}=\sqrt{4πn_ee^2/m_e },$ where $e$ and $m_e$ are the charge and mass of the electron; here and below, the formulas use the Gaussian system of units (GSS); Temperature in P. physics is usually measured in energy. units (1 keV≈107 K). The spatial and temporal scales are usually small, so the concentrations of positive and negative charges turn out to be almost the same $(|Z_{eff}n_i-n_e|/n_e≪1)$; in this sense, they speak of the quasi-neutrality of P. This most important property of P. is often used to determine P., following I. Langmuir, who first used it in the 1920s. the term "P." to designate the quasineutral region of the gas discharge remote from the electrodes. Typically, the lifetime and size of a particle exceed $r_D$, respectively, which ensures its quasi-neutrality. P.'s quasineutrality does not contradict the presence of volumetric electricity. fields in a P. located in a magnetic field.
Classification of plasma types
The classification of plasma types is conditional. If there are many charged particles in a sphere of radius $r_D$ ($N≈4πnr_D^3/3≫1, n$ is the concentration of all plasma particles), the plasma is called an ideal plasma; for $N⩽1$ we speak of a nonideal plasma (here $N$ is the ideality parameter). In an ideal planet, the potential energy of interaction between particles is small compared to their thermal energy.
Highly ionized plasma with a temperature of $⩾10^2–10^3$ eV is called high-temperature plasma, in contrast to low-temperature plasma with $T_e⩽10–100$ eV, in which collisional and radiation processes can play a significant role. A special type of low-temperature plasma is dust plasma, which contains macroscopic (from fractions to hundreds of micrometers in size) solid particles that carry a large electric current. charge $(Z_{eff}≫1)$. A high-temperature P. with high electrical conductivity $σ$ is also called ideal if dissipative processes can be neglected.
At ultra-high energy densities resulting from collisions of heavy ultrarelativistic particles, the formation of quark-gluon plasma is possible—a hadronic medium in which the colored charges of quarks and gluons are mixed, just as electric charges are mixed in a conventional plasma. charges. Cryogenic plasma particles (with a temperature of a fraction of a kelvin) are created by precision ionization of cold atoms with a laser beam, the energy of the quanta of which is almost equal to the ionization energy. To describe electrons in metals, the charge of which is compensated by the charge of crystalline ions. lattices, as well as electrons and holes in semiconductors, the term plasma of solids is often used. Modern P. physics also considers laser plasma that occurs during optical breakdown under the influence of powerful laser radiation on a substance; charged plasma, in particular electron and ion beams, charged layers (electric double layer), etc.
A particle is called degenerate at a low temperature $T$ and a high concentration of particles $n$, when the characteristic distance $(∝n^{–1/3})$ between them becomes of the order of the de Broglie wavelength $(λ≈h/ (2mT)^{1/2}$, where $h$ is Planck’s constant). Artificially created P. is usually thermodynamically nonequilibrium. Local equilibrium occurs only if particles collide with each other. Equilibrium is established most quickly within the electron component of the electron, and in the ionic component and between ions and electrons, it is $\sqrt{∼m_i/m_e}$ and $∼m_i/m_e$ times slower, respectively. Unlike gas, the frequency of particle collisions decreases with increasing particle energy ($∝T^{–3/2}$). Based on the number of types of ions, single-component and multicomponent plasmas are distinguished.
Plasma in nature and technology
It is believed that more than 99% of the baryonic matter in the Universe is in the P. state in the form of stars, interstellar and intergalactic matter. gas (see Cosmic plasma). The power of the magnetosphere protects the Earth from the destructive flow of power emitted by the Sun—the solar wind. The presence of ionospheric radiation, which reflects radio waves, makes long-distance radio communication possible. Lightning in nature can be observed in the form of atmospheric discharges (lightning and corona discharges) and auroras, as well as in ordinary flames. In technology, the most widespread is the use of gas discharges, used in laboratory and technological applications. purposes, in gas-discharge light sources (for example, fluorescent lamps), in switching devices, when welding and cutting materials, in plasma panels of television and multimedia screens. P. flows are used in plasmatrons for processing materials, in surgery, and in plasma space applications. engines and magnetohydrodynamic generators. In high-temperature P. thermonuclear reactions can occur. To implement controlled thermonuclear fusion (CTF) in a deuterium-tritium plasma, it is necessary to fulfill the Lawson criterion—containment of the plasma with $T⩾10$ keV and $n⩾10^{14}$ cm–3 for a time of $⩾1$ s (in P. other compositions these values are even higher). Typical parameter values dec. types of plasma are shown in the figure.
Viscosity
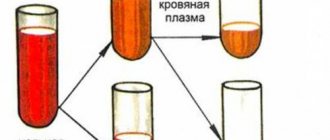
Blood viscosity is its ability to resist the flow of fluid during the movement of particles using internal friction. On the one hand, these are complex relationships between macromolecules of colloids and water, on the other, between formed elements and plasma. The viscosity of plasma is higher than that of water. The more large molecular proteins it contains (lipoproteins, fibrinogen), the stronger the viscosity of the plasma. In general, this property of blood is reflected in the general peripheral vascular resistance to blood flow, that is, it determines the functioning of the heart and blood vessels.
Methods for describing plasma
Ranges of parameters of various types of plasma. Straight lines delimit areas of ideal and non-ideal plasma (ideality parameter N=1), areas of classical and degenerate plasma (T=ℰF, ℰF –…
The natural way to describe a particle by calculating the motion of all its particles cannot be implemented in practice even with the help of powerful calculations. technology due to the collective nature of particle interaction. However, many important properties of P. can be understood on the basis of analysis of the movement of parts. particles. In a magnetic field with induction $\boldsymbol B$, the movement of charged particles along and across the direction of the magnetic field is significantly different. In the longitudinal direction, a particle with a charge $q$ moves translationally, and in the transverse direction it rotates with a cyclotron frequency $ω_B=qB/mc$ ($c$ is the speed of light). If the Larmor radius $ρ_L=v_⟂/ω_B$ of such rotation is less than the mean free path of the particle and the characteristic size of the particle, and the electromagnetic field changes slowly compared to the period of cyclotron rotation, the particle is considered to be a magnetized plasma ($v_⟂$ is the speed of the particle across the magnetic field). Particles of such a particle move while maintaining adiabatic motion. invariant – magnetic moment $μ \approx mv_⟂^2/2B$, and under the influence of the k.-l. forces $\boldsymbol F$, the Larmor spirals they describe slowly drift across the magnetic field with a speed $\boldsymbol v_F=c[\boldsymbol F×\boldsymbol B]/qB^2$. Depending on the nature of the force $\boldsymbol F$, gravitational, electric, gradient, centrifugal and polarization drifts are distinguished (see Drift of charged particles). The direction of cyclotron rotation of particles is determined by Lenz's rule: the magnetic field of the current of cyclotron rotation of particles is opposite to the external field and, therefore, weakens it. This manifests itself in the diamagnetism of the magnet, which leads to its expulsion from the region of a stronger magnetic field.
The identity of the particles of each component of P. allows the use of kinetic. description using a single-particle distribution function $f(t, \boldsymbol r, \boldsymbol v)$, defined as the concentration of particles of a given component in phase space (see Kinetic equations for plasma). Like the usual concentration, the distribution function satisfies the continuity equation, but only in phase space: $