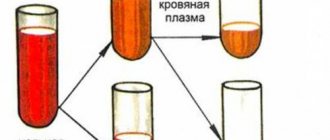
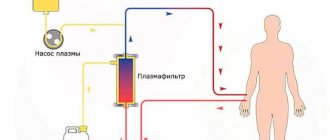
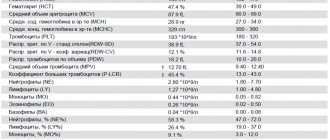
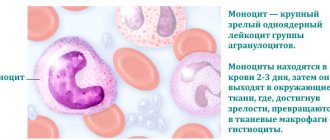
Equations [edit | edit code]
Let a be the radii of the circles, the origin of coordinates is at the rightmost point of the horizontal diameter of the fixed circle (see figure). Then the cardioid equations can be written in the following forms [2]:
- In rectangular coordinates[1]: ( x 2 + y 2 + 2 ax ) 2 − 4 a 2 ( x 2 + y 2 ) = 0 +y^2>+2ax)^2>-4a^2>(x^ 2>+y^2>),=,0>2>
- In rectangular coordinates (parametric notation): x = 2 a cos t − a cos 2 ty = 2 a sin t − a sin 2 t
- In polar coordinates[2][1]: r = 2 a ( 1 − cos φ )
Properties of a Linear Function
- The domain of a function is the set of all real numbers.
- The set of values of a function is the set of all real numbers.
- The graph of a linear function is a straight line. To construct a straight line, it is enough to know two points. The position of the straight line on the coordinate plane depends on the values of the coefficients k and b.
- The function has neither the largest nor the smallest values.
- Evenness and oddness of a linear function depend on the values of the coefficients k and b: b ≠ 0, k = 0, which means y = b is even; b = 0, k ≠ 0, which means y = kx is odd; b ≠ 0, k ≠ 0, which means y = kx + b is a function of general form; b = 0, k = 0, which means y = 0 is both an even and an odd function.
- A linear function does not have the property of periodicity, because its spectrum is continuous.
- The graph of the function intersects the coordinate axes: the abscissa axis OX is at the point (-b/k, 0); The ordinate axis OY is at point (0; b).
- x=-b/k is the zero of the function.
- If b = 0 and k = 0, then the function y = 0 vanishes for any value of the variable x. If b ≠ 0 and k = 0, then the function y = b does not vanish for any value of the variable x.
- The function increases monotonically on the domain of definition for k > 0 and decreases monotonically for k < 0.
- When k > 0: the function takes negative values on the interval (-∞, -b/k) and positive values on the interval (-b/k, +∞) When k < 0: the function takes negative values on the interval (-b/k , +∞) and positive values on the interval (-∞, -b/k).
- The coefficient k characterizes the angle formed by a straight line with the positive direction Ox. Therefore k is called the slope. If k > 0, then this angle is acute; if k < 0, it is obtuse; if k = 0, then the straight line coincides with the Ox axis.
There are two special cases of a linear function:
- If b = 0, then the equation will take the form “y = kx”. This function is called direct proportionality. A graph is a straight line that passes through the origin.
- If k = 0, then the equation becomes “y = b”. The graph is a straight line that is parallel to the Ox axis and passes through the point (0; b).
Properties [edit | edit code ]
- Cardioid is a special case of Pascal's cochlea
- A cardioid is a special case of a sinusoidal spiral
- Cardioid is an algebraic curve of the fourth order.
- The cardioid has one cusp.
- The arc length of one turn of the cardioid, given by the formula in polar coordinates
r = 2 a ( 1 − cos φ ) is equal to: L = 2 ∫ 0 π r ( φ ) 2 + ( r ′ ( φ ) ) 2 d φ = ⋯ = 8 a ∫ 0 π 1 2 ( 1 − cos φ ) d φ = 8 a ∫ 0 π sin ( φ 2 ) d φ = 16 a ^+(r'(varphi ))^2>>>;dvarphi =cdots =8aint _2>0>^>(1- cos varphi )>>;dvarphi =8aint _2>1>0>^sin(>)dvarphi =16a>
- Area of a figure bounded by a cardioid given by the formula in polar coordinates
r = 2 a ( 1 − cos φ ) is equal to: S = 2 ⋅ 1 2 ∫ 0 π ( r ( φ ) ) 2 d φ = ∫ 0 π 4 a 2 ( 1 − cos φ ) 2 d φ = ⋯ = 4 a 2 ⋅ 3 2 π = 6 π a 2 1>2>>int _2>0>^>;dvarphi =int _2>0>^(1-cos varphi )^2>>;dvarphi =cdots =4a ^2>cdot 3>2>>pi =6pi a^2>>.
Radius of curvature of any line:
ρ ( φ ) = [ r ( φ ) 2 + r ˙ ( φ ) 2 ] 3 / 2 r ( φ ) 2 + 2 r ˙ ( φ ) 2 − r ( φ ) r ¨ ( φ ) .
2>+>(varphi )^2> ight]^>+2>(varphi )^2>-r(varphi )>(varphi )>> .>
What gives for a cardioid given by an equation in polar coordinates:
r ( φ ) = 2 a ( 1 − cos φ ) = 4 a sin 2 φ 2 ,
2>2>>,> ρ ( φ ) = ⋯ = [ 16 a 2 sin 2 φ 2 ] 3 2 24 a 2 sin 2 φ 2 = 8 3 a sin φ 2 . sin ^2>2>>]^3>2>>>sin ^2>2>>>>=>asin 3>8>2>> .>
Construction of a linear function
There is an axiom in geometry: through any two points you can draw a straight line, and only one. Based on this axiom, it follows: to build a graph of a function of the form “y = kx + b”, it is enough to find only two points. And to do this, you need to determine two x values, substitute them into the function equation and calculate the corresponding y values.
For example, to plot the function y = 1/3x + 2, you can take x = 0 and x = 3, then the ordinates of these points will be equal to y = 2 and y = 3. We get points A (0; 2) and B (3 ; 3). Let's combine them and get the following graph:
In the equation of the function y = kx + b, the coefficient k is responsible for the slope of the function graph:
- if k > 0, then the graph is tilted to the right;
- if k < 0, then the graph is slanted to the left.
Coefficient b is responsible for shifting the graph along the OY axis:
- if b > 0, then the graph of the function y = kx + b is obtained from y = kx with a shift of b units upward along the OY axis;
- if b < 0, then the graph of the function y = kx + b is obtained from y = kx with a shift of b units down along the OY axis.
Let's draw three graphs of the function: y = 2x + 3, y = 1/2x + 3, y = x + 3.
Let's analyze the drawing. All graphs are skewed to the right because in all functions the coefficient k is greater than zero. Moreover, the higher the value of k , the steeper the straight line goes.
In each function b = 3, so all graphs intersect the OY axis at point (0; 3).
Now consider the graphs of the functions y = -2x + 3, y = -1/2x + 3, y = -x + 3.
This time, in all functions the coefficient k is less than zero, and the graphs of the functions are tilted to the left. The larger k , the steeper the straight line.
The coefficient b is three, and the graphs also intersect the OY axis at (0; 3).
Consider the graphs of the functions y = 2x + 3, y = 2x, y = 2x - 2.
Now in all function equations the coefficients k are equal. We got three parallel lines.
In this case, the coefficients b are different, and these graphs intersect the OY axis at different points:
- the graph of the function y = 2x + 3 (b = 3) intersects the OY axis at point (0; 3);
- the graph of the function y = 2x (b = 0) intersects the OY axis at the origin (0; 0);
- the graph of the function y = 2x - 2 (b = -2) intersects the OY axis at point (0; -2).
Lines will be parallel when their angular coefficients coincide.
Let's summarize. If we know the signs of the coefficients k and b , then we can imagine what the graph of the function y = kx + b looks like.
If k < 0 and b > 0, then the graph of the function y = kx + b looks like this:
If k > 0 and b > 0, then the graph of the function y = kx + b looks like this:
If k > 0 and b < 0, then the graph of the function y = kx + b looks like this:
If k < 0 and b < 0, then the graph of the function y = kx + b looks like this:
If k = 0, then the function y = kx + b is converted to the function y = b. In this case, the ordinates of all points on the graph of the function are equal to b. And the graph looks like this:
If b = 0, then the graph of the function y = kx passes through the origin. This is what a direct proportionality graph looks like:
In 7th grade problems you can find a graph of the equation x = a. It is a straight line that is parallel to the OY axis, all points of which have the abscissa x = a.
It is important to understand that the equation x = a is not a function, since different values of the argument correspond to the same value of the function, which does not correspond to the definition of a function.
For example, the graph of the equation x = 3:
Condition for parallelism of two lines:
The graph of the function y = k1x + b1 is parallel to the graph of the function y = k2x + b2 if k1 = k2.
The condition for the perpendicularity of two straight lines:
The graph of the function y = k1x + b1 is parallel to the graph of the function y = k2x + b2 if k1 * k2 = -1 or k1 = -1/k2.
Points of intersection of the graph of the function y = kx + b with the coordinate axes:
- With OY axis. The abscissa of any point that belongs to the OY axis is equal to zero. Therefore, to find the point of intersection with the OY axis, you need to substitute zero in the equation of the function instead of x. Then we get y = b. Coordinates of the point of intersection with the OY axis: (0; b).
- With OX axis. The ordinate of any point that belongs to the OX axis is equal to zero. Therefore, to find the point of intersection with the OX axis, you need to substitute zero in the equation of the function instead of y. And we get 0 = kx + b. So x = -b/k. Coordinates of the point of intersection with the OX axis: (-b/k; 0)
History [edit | edit code]
The cardioid was first encountered in the works of the French scientist Louis Carrè
, 1705).
The name of the curve was given in 1741 by Giovanni Salvemini di Castillone (he is also referred to as Johann Francesco Melchiore Salvemini Castillon
).
"Straightening", that is, the calculation of the length of the curve, was performed by La Hire ( Philippe de La Hire
), who discovered the curve independently, in 1708.
Koersma
(1741) also independently described the cardioid Subsequently, many prominent mathematicians of the 18th–19th centuries showed interest in the curve.
One and one makes two. Everyone is lonely - here you are, and there I am. People are always doubly lonely when they are alone with themselves. If something could bring them together, the two would immediately become one. Let mathematics combine our hearts to take us all the way to the end.
Williams Jay, "Heroes of Nowhere"
The post probably should have been titled "How to Draw an Animated Valentine's Day Heart Using Math in an Inappropriate Way." I rejected this name in favor of a more poetic one: after all, a wonderful romantic holiday is approaching, which we, IT specialists and other nerds, must meet fully armed. I will immediately show you the result, and under the hack there will be a lot of letters about how I achieved this result.
Disclaimer
I realize that a beautiful blinking heart can be made without the slightest knowledge of mathematics. But is this interesting?
Step 1. Parameterize the heart.
First, we need a mathematical object that at least vaguely resembles a heart. Fortunately, for me this step was trivial: a couple of years ago I discovered a wonderful formula for just such a case (for aesthetic reasons, the graph in the figure is stretched horizontally, in fact it should fit between -1 and 1).
The formula was discovered from the following considerations: take an ordinary circle and imagine that it consists of jelly, while being rigidly attached to the ordinate axis. Now let’s “blow” on it from below: add to the Y coordinate a certain function w(x) = w(x(t)), equal to zero at x=0, monotonically increasing at x>0 and even in x. After such a “blow,” the halves of the circle will move upward, forming “bulges” of the heart, and thanks to the rigid attachment to the Y axis, a lower “tail” and an upper “dent” are formed. In this case w(x(t)) = |x| 1/2 = |cos(t)| 1/2. You can try another “blowing function” yourself and see what happens.
Step 2. From parametric specification to implicit function.
x = cos(t) y = sin(t) + |cos(t)| 1/2 y - |x| 1/2 = sin(t) (y - |x| 1/2 ) 2 + x 2 = 1 f(x,y) = (y - |x| 1/2 ) 2 + x 2 - 1 = 0
Step 3. From an implicit function to a function of two variables. Function of color.
With f(x,y) in hand, we can finally realize our dream: to draw a beautiful color picture. To do this, we need one more function: the color function. It should take a real argument r and return an integer value between 0 and 255. It is also desirable that it be monotonic on each semi-axis and have a maximum at zero. As such a function, you can take, for example, this one:
Here 100 is the “magic number”; later, in full accordance with “good programming style,” we will replace it with a parameter. Now for each point (x,y) we can set the color as rgb(c(f(x,y)), 0, 0). Those points that previously belonged directly to the “heart” graph have become bright red (note the stationary light outline in the GIF). As you move away from the graph, the color will fade until it becomes black at some distance from it.
Step 4. Add a parameter and create an animation.
Now let's replace the magic number 100 with the parameter k. The new color function looks like this:
Let k be some function of time. Then for each point in the image at each moment in time we can calculate its color (which is, in fact, the mathematical definition of animation). At first I wanted to take something like k(t) = 80(sin(t)+1). Then, however, I realized that with a large number of frames, the GIF would weigh more than 640 kilobytes. On the other hand, with a small number of frames there is no point in bothering with the analytical task k(t). In the end, to get the heart pulsating, I sequentially assigned k values of 80, 90, 100, 110, 120, 110, 100, 90, and then combined the images generated for these values into a looping GIF. In general, that's all.
Concept of function
A function is a dependency of "y" on "x", where "x" is the variable or argument of the function and "y" is the dependent variable or value of the function.
To define a function means to define a rule according to which the corresponding values of the independent variable can be found. Here are the ways you can set it:
- Tabular method - helps to quickly determine specific values without additional measurements or calculations.
- Graphic method - visually.
- The analytical method is through formulas. It is compact, and you can calculate the function for an arbitrary value of the argument from the domain of definition.
- Verbal method.
The graph of a function is the union of all points, when instead of “x” you can substitute arbitrary values and find the coordinates of these points.
Ideal heart. Note to mathematicians
From a lecture on anatomy, for example.
Let me explain: normally a person’s pulse changes. Always. Heart rate changes + - several beats per minute every few seconds - get yourself a constant meter and you will understand what I mean. Just 2 weeks ago I measured it for 2 days, so believe me, I know what I’m talking about.
In the event of a heart attack, the first pacemaker (I may be a little confused in the terminology, I’ve never been on good terms with it) is switched off, the second (secondary) works, which sets an exceptionally even pulse, no longer dependent on the state of the body) There is also a third- th, tertiary, pacemaker (I forgot what system it is), but it cannot maintain a pulse above 30 beats per minute, and therefore, when it works, the person usually lies unconscious. This is if the second one, more stable, failed. III is almost invulnerable on its own.
I'm telling you, it's good. I believe
Thank you, comrade doctor, but for me this is already like meat against the wall)) I’m not a doctor ((If I just look at the finished cardiogram, maybe I’ll figure it out. In fact, I think it would be worth practicing, but I haven’t had to yet due to my studies. Not the right direction )
Yes exactly. These nodes and bundle of His.
5 + (-sqrt(1-x^2-(y-abs(x))^2))*cos(30*((1-x^2-(y-abs(x))^2))) , x is from -1 to 1, y is from -1 to 1.5, z is from 1 to 6
(x^2+(9 y^2)/4+z^2-1)^3-x^2 z^3-(9 y^2 z^3)/80 = 0
A mathematical model has been created that allows us to find out the fate of a person
Russian scientists have derived a mathematical formula for the heart. Thanks to these equations, any heart disease can be calculated, predicted and prevented. That is, in essence, to find out and change a person’s fate. The only laboratory of mathematical physiology in Russia operates at the Yekaterinburg Institute of Immunology and Physiology. Corresponding Member of the Russian Academy of Sciences Vladimir Markhasin has been engaged in mathematical developments in the “core” field for 25 years.
“The problem of mathematical descriptions of the physiological functions of the body is the second most important problem after the problem of human DNA,” says Vladimir Semenovich. – In the future, formulas for other human organs will be calculated, and doctors, using elementary equations, will be able to predict and treat any disease!
“A person, in fact, is a complex mechanism in which physical and chemical processes continuously occur,” continues the mathematician. - If all these processes are translated into the language of equations, then in the future it will be possible to derive a single formula for man!
Functions and their graphs
Functions are mathematical portraits of stable patterns cognizable by humans. To illustrate the characteristic properties of functions, let us turn to proverbs and sayings. After all, proverbs are also a reflection of stable patterns, verified by the centuries-old experience of the people.
“The further into the forest, the more firewood”
. The graph will present the amount of firewood as a function of the path [6].
“You can’t spoil porridge with butter.”
The quality of porridge can be considered a function of the amount of oil in it. According to the proverb, this function will not decrease with the addition of oil. It may increase, but it may remain at the same level [5].
Functions in economics
Graphs are widely used in economics, in particular, the supply and demand curve, and the production possibilities line.
Over the past few months, countries around the world have been in a state of financial and economic crisis that began in the United States. The crisis also came to Russia. We were interested in what functional dependencies in the economy have undergone changes in connection with this, and how. Mathematical economics studies these issues. —
a science whose subject is mathematical models of economic objects and processes.
Economic growth in Russia in the early 2000s was largely determined by high prices for energy resources: oil and gas. And when oil prices fell, the flow of money to Russia decreased. As a consequence of this, domestic demand for products decreased, which in turn led to a reduction in production. The financial crisis turned into an industrial crisis [8].
Modern mathematics knows many functions, and each has its own unique appearance, just as the unique appearance of each of the billions of people living on Earth is unique.
The appearance of each function can be imagined as composed of a set of characteristic details. They reveal the basic properties of functions.
Observing various processes and phenomena, we try to discern their most significant features, the deepest patterns. They often turn out to be common to a wide range of observed events. The mathematical model built on the basis of these laws also turns out to be common.
.
Conclusion
The concept of function is one of the basic concepts of mathematics in general. It did not arise immediately in the form in which we use it now, but, like other fundamental concepts, it has gone through a long path of historical development. The idea of functional dependence dates back to ancient Greek mathematics.
The term “function” was first introduced into consideration by the famous German mathematician and philosopher Leibniz in 1694. However, he uses this term (he did not give a definition at all) in a narrow sense, understanding by function a change in the ordinate of a curve depending on the change in its abscissa. Thus, the concept of function has a “geometric character” for him.
Work and power of the heart. Heart-lung machine
The work done by the heart is spent on overcoming pressure forces and communicating kinetic energy to the blood.
Let's calculate the work done during a single contraction of the left ventricle.
Let's depict Vу
- stroke volume of blood - in the form of a cylinder (Fig. 9.9).
We can assume that the heart pushes this volume along the aorta with a cross-section S
to a distance
l
at an average pressure
p.
The work done
Work is required to impart kinetic energy to this volume of blood
where r is blood density, u
- blood speed in the aorta. Thus, the work of the left ventricle of the heart during contraction is equal to
Since the work of the right ventricle is taken to be equal to 0.2 of the work of the left, then the work of the entire heart during a single contraction
Formula (9.17) is valid for both rest and the active state of the body. These conditions are distinguished by different blood flow rates.
Substituting into formula (9.17) the values
p
= 13 kPa,
Vy =
60 ml = 6 • 10 -5 m 3, r = 1.05 • 10 3 kg/m 3,
u =
0.5 m/s, we obtain the work of one-time contractions of the heart at rest:
Al »
1 J. Assuming that on average the heart makes one contraction per second, we find the work of the heart per day:
Ac
= 86,400 J. With active muscular activity, the work of the heart can increase several times.